Calculation of stress intensification factors

PASS/NOZZLE-FEM 3.5. Program Manual

5.6. Calculation of stress intensification factors (SIF)

The stress concentration factor (SCF) is defined as follows:

$$ SCF = \frac{K_m \sigma_{MAX}}{\sigma_{NOM}} $$

(5.12)

where	$\sigma_{MAX}$	-	maximum secondary stress (secondary membrane plus bending, including thermal expansion), that is determined from finite element analysis (FEA);
	$\sigma_{NOM}$	-	nominal stress depending on rule codes and loading type.

The peak stress $\sigma_{NOM}$ is based on the secondary stress (secondary membrane plus bending, including thermal expansion) taking into account stress intensification in the weld:

$$ \sigma_{PEAK} = \frac{K_f}{i_w}\sigma_{MAX} $$

(5.13)

where	$K_f$	-	fatigue strength reduction factor (FSRF), by default is equal to 1.35;
	$i_w$	-	intensification factor for a transverse weld between pipes, by default is equal to 2.00.

The stress intensification factor $i$ is calculated as the ratio of peak stresses to nominal:

$$ i = \frac{\sigma_{PEAK}}{\sigma_{NOM}} = \frac{K_f}{i_w} SCF $$

(5.14)

Fatigue strength reduction factor $K_f$ is a stress intensification factor which accounts for the effect of a local structural discontinuity (stress concentration) on the fatigue strength. It is the ratio of the fatigue strength of a component without a discontinuity or weld joint to the fatigue strength of that same component with a discontinuity or weld joint. Values for some specific cases are empirically determined (e.g., socket welds). In the absence of experimental data, the stress intensification factor can be developed from a theoretical stress concentration factor derived from the theory of elasticity or based on the guidance provided in Tables 5.11 and 5.12 [4].

Stress intensification factors are defined for runhead pipe and bend (elbow) leg, and also for nozzle juncture. Depending on selection: element's edge or junction place, will be produced different values for the nozzle.

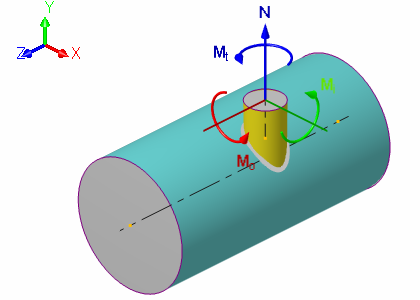Fig. 5.29. Local coordinate system for SIF calculation

Table 5.5 shows the calculation of the stress intensification factors for local coordinate system of nozzle (fig. 5.29).

Table 5.5. Stress intensification factors (SIF)
Description		Fatigue strength reduction factor	Stresses, MPa		Stress concentration/intensification factors
		Fatigue strength reduction factor	Max.	Nom.	Stress concentration/intensification factors
		K_f	σ_MAX	σ_NOM	SCF	i (SIF)
From axial force N	i_a	1,3500	1050,2615	77,7000	14,5306	9,8082
From inplane moment M_i	i_i	1,3500	276,9120	77,7000	3,8311	2,5860
From outplane moment M_o	i_o	1,3500	667,5290	77,7000	9,2354	6,2339
From torsion moment M_t	i_t	1,3500	252,0132	77,7000	3,4867	2,3535
From design pressure p	i_p	1,3500	345,7572	77,7000	4,4499	3,0037

PASS/NOZZLE-FEM 3.5. Program Manual